Introduction to Harmonics
Harmonic procedures build quality/quantity, string-length/frequency, space/time, philosophic/scientific, sound/haptic/visual, and more, sensibilities. Harmonics is especially suited but not limited to the Arts and Sciences. This includes all the disciplines and therefore also applications to the Humanities and including seemingly far fetched outside disciplines such as Economics or Law. Each has its Harmonic Measure to be infered properly by their practitioners. The study of Harmonics will inhance a persons understanding for Harmonic Measure within ones discipline. Harmonic data may be exchanged in an interdisciplinary manner by those who have mastered the basics of Harmonics. From this point the discussion may begin between people from different disciplines. The stockbroker-harmonist and the zoologist-harmonist are checking resources, the physicist-harmonist and astronomer-harmonist exchange tones, while the artist-harmonist sees how this expansion might engage a World Harmonics - a Unified Standard for interdiscipinary observation and exchange for everything from crayons and nanoseconds to string theory. Let's exchange the word theory for standard when talking about a unified system. A good reason to adopt the standard of Harmonics as a universal lies in the natural reciprocity of string length to frequency which means that what you see you can also hear. For every quantity a quality (tone) can be percieved. This ranks among the earliest findings of humans. With this tool, as vibration turns into light, these frequencies can be octave reduced into the range of hearing and demonstrated on a string length or at current frequency resolution on a computer. This is the essence of the harmonic communication which to attain, one must practice with some determination until fluent; not unlike a musical instrument, but with a seeing and hearing listening. Studying Harmonics one learns about the interconnectivity between all disciplines. Discovered through experiencing ratio and proportion as sound and sight. This experience is also achieved through harmonic procedures such as hands-on work with one string length, otherwise known as a monochord, mixing color drops into tables, discovering number theory, harmonic drawing and also outdoors on a green expansive lawn with stakes and rope for perspective projections. Over time this practice lights into the fabric of living, a plan for sharing and comparison of occurrence and structure between disciplines. Unified! Standard.
Students with computer programming experience or those involved with gathering DATA in any field stand to benefit from the humanist/naturalist emphasis harmonic applications place on the hapitcal/codifying way Harmonics works upon intuition, construction and aesthetics. A computer is not necessary for harmonic evaluations while learning Harmonic principles, but can later do much to organize harmonic thought for communication and the creation of new work. The primary tool for learning Harmonics is the monochord. This in no way limits the study to its usage. Numerical and visual examples also prove harmonic theorems.
The principles of Harmonics were discovered by Pythagoras c.587-c.507 B.C. during travels to Egypt and throughout the ancient world. Pythagoras first began to teach at the age of 50. His theories were first introduced to the western world in Plato's Timeaus, Chapter 6, The Soul of the World in the form of the Tetraktys. A translation in the 15th Century spurred on the Renaissance. It resurfaced during the 16th century with the astronomer Kepler and further with Newton in the 17th Century. The work of the great 19th century physicist Hermann Helmholz applied the scientific method to tone and fueled a misconception that Microtonality and Harmonics are the same discipline. At about the same time as Helmholz, Albert Thimus a native of Cologne, Germany translating latin and greek texts rediscovered the Lambdoma which is the basis for Harmonics. Based on Thimus, Harmonic Symbolism in Antiquity - published 1866 (Harmonikale Symbolik des Alterthums), Hans Kayser a student of Thimus, also from Cologne, wrote a series of works, that organize and demonstrate Harmonic principles. His most important work is the Handhook of Harmonics - Zurich 1950 (Lehrbuch der Harmonik). This work organizes Harmonics for a formal study. Fields examined by Kayser include but are not limited to: philosophy, mathematics, astronomy, architecture, grammar, crystallography, color mixing, botany and microtonality. From this perspective microtonality is in the domain of Harmonics. The lineage is also clear with composer Harry Partch being one of the first practicing harmonists in the 20th Century when new Pythagoreans surfaced within the Arts and Sciences.
The main focus of Harmonics is the study of a three dimensional model called the Lambdoma. The Lambdoma is constructed by combining the ratios of the arithmetic, harmonic and geometric series into one form. Ernest G. McClain (USA) in his book The Pythagorean Plato - Nicholas-Hays, York Beach, Maine 1978, confirmed that the Lambdoma was recognized in antiquity by findings that were concealed within the Marriage Allegory of Plato's Republic.
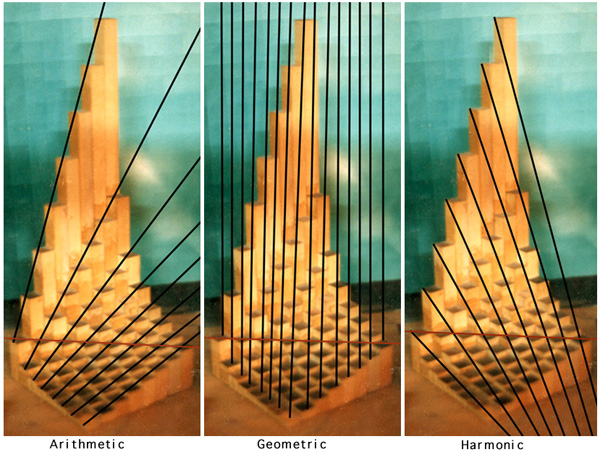
An indexed 8 Lambdoma is shown here. In theory the index is unlimited. The red lines show the 1/1 ratio in each of the three representations. To one side all ratios are greater than 1/1 and to the other less than 1/1; all on the green color drop lambdoma background.
Mans ability to invent and develop language and number assured a high degree of sophistication for communication and clarifying phenomena. What is the basis for the impulse that drives an individual's action? One most appropriate answer would be choice. Choice suggests a use of language, an action and in the broadest sense, a setting of standards. Each choice becomes part of a series of events comprising periods of time that collectively describe experience.
In any situation you might be bid. Give me one! It is presumed that you understand a 'unit' as the circumstance demands. Each discipline has its own unique set of units or standards that we may use for communication. It is much easier to use available standards than create new ones. Still, gaps exist between disciplines that limit communication. It could prove useful to have a standard that provides a unit for exchange. Such a system would need to possess universal standards. It is quite easy to add apples and oranges, as long as apple remains apple per unit orange or otherwise. The same principle holds graphically, when changing for example certain black pixels red in a color map. Here reciprocity provides a means for non-conventional comparison. This thought is the basis for interdisciplinary communication and the standard making all this possible lies in the application of Harmonics.
The fabric of Harmonics is ratio and proportion. In elementary mathematics we are introduced to fractions and some of the following different ways of saying them and their reciprocals: one half, one to two, one divided by two, one part and two parts, two divided by one, two to one and further to the singular two or one. Harmonic ratios are referenced best as expressions of unity such as two to three (2/3 or 3/2). This reference aims at a comparison of unity or amounts of one element to another. The selection of elements can be quite tricky because we do not normally compare apples and oranges. The use of a computer allows these comparisons in the DATA world with both graphic and sound outputs. Probing with instruments is dependent upon the factor of resolution. We'll continue to separate theory and standard. Now would be a good time to show the particulars of Harmonics in an outline form based upon Hans Kayser's Handbook of Harmonics.
In any situation you might be bid. Give me one! It is presumed that you understand a 'unit' as the circumstance demands. Each discipline has its own unique set of units or standards that we may use for communication. It is much easier to use available standards than create new ones. Still, gaps exist between disciplines that limit communication. It could prove useful to have a standard that provides a unit for exchange. Such a system would need to possess universal standards. It is quite easy to add apples and oranges, as long as apple remains apple per unit orange or otherwise. The same principle holds graphically, when changing for example certain black pixels red in a color map. Here reciprocity provides a means for non-conventional comparison. This thought is the basis for interdisciplinary communication and the standard making all this possible lies in the application of Harmonics.
The fabric of Harmonics is ratio and proportion. In elementary mathematics we are introduced to fractions and some of the following different ways of saying them and their reciprocals: one half, one to two, one divided by two, one part and two parts, two divided by one, two to one and further to the singular two or one. Harmonic ratios are referenced best as expressions of unity such as two to three (2/3 or 3/2). This reference aims at a comparison of unity or amounts of one element to another. The selection of elements can be quite tricky because we do not normally compare apples and oranges. The use of a computer allows these comparisons in the DATA world with both graphic and sound outputs. Probing with instruments is dependent upon the factor of resolution. We'll continue to separate theory and standard. Now would be a good time to show the particulars of Harmonics in an outline form based upon Hans Kayser's Handbook of Harmonics.
• • •
I. Introduction - History of Harmonics.
II. Theorems of Tone Numbers.
II. Theorems of Tone Numbers.
A. Use of the monochord.
B. Fractions, decimals, base 2 logarithms, cents, frequency, polar coordinates, tone angles, string lengths and tone values.
C. Reciprocity of frequency and string length, the geometric arrangement of time and space.
D. Equality within musical proportions - principles of equal distance.
E. Acoustics - rhythm, periodicity, resonance and interference.
F. The state of simultaneous conflicting attitudes; continua: right/left; material/psychic; major/minor; static/dynamic and so forth.
G. Examination of the senses and an analogy of scientists probing blindly with instruments - reliability and resolution.
III. Theorems of Tone Rows.
A. The overtone row, undertone row, Plato's tetraktis.
B. Whole numbers.
C. The intervals, octave multiplication and reduction.
D. Calculating base 2 logarithms, the number 'e', the I Ching, Weber-Fechner law.
E. Convergence, divergence, perspective, symmetry.
1. The rows of Archimedes and Leibnitz.
2. The straight and the curved.
3. Reciprocal relationship of the eye and ear,
(equal distance & perspective).
IV. Theorems of Tone Groups.
A. The lambdoma of Albert von Thimus. The partial tone diagram - interpolation of overtone and undertone rows ("T" diagrams & the rationing of coordinates).
B. Major & minor tone rows, Thimus rows, the binomial theorem.
C. Polarity - further discussion of reciprocity. A three dimensional model of the partial tone diagram (lambdoma).
D. Equal tone lines, harmonic rays - tones with the same character but different degrees (3/1, 3/2, 3/4...) or identical tones (2/1, 4/2, (6/3...)
E. Index and generators of the partial tone diagram.
F. Parabolas, hyperbolas and ellipsis (conic sections) as derived from the partial tone diagram.
G. Arithmetic, harmonic and geometric proportions (partial tone diagram). Irrational numbers comprehended geometrically. Harmonic number as a mediator between concept, idea and sensuality (Haptik).
H. Harmonic proportions in architecture.
I. Trinities: 1/n - 1/1 - n/1; morning - noon - evening; right - middle - left, (world religions).
V. Structure of Tone Groups.
A. Variations of the partial tone diagram (triangular, quadratic and circular).
B. Combinations of three variation forms (triangular, quadratic and circular).
C. Polar diagrams - the circle as a monochord sl:ring divided by whole numbers.
D Tone spirals and tone curves, logarithmic and decimal - the tone cycloid, planets and orbits, a proof for irregularities in the harmonic system and a further discussion of perspective and reciprocity.
E.- The completed partial tone diagram - coordinates in four directions (not considered as a combination type). Examination of the free fall law of Galileo and Newton's gravity law as compared with the consistency of time and space in perspective and equal distant proportion. A discussion concerning the concept of gestalt.
F. Logarithmic structure - a logarithmic graph of the completed partial tone diagram.
G. Tonal space - a cubic model made from partial tone diagrams, the three dimensional spiral (screw), tone sphere, platonic solids, other examples from crystallography, discussion of the multifunctionality of the ear.
H. Sound Pictures.
1. A discussion of seeing and hearing - geometric form and bridging gaps between disciplines comparing the Wolfram model.
2. Harmonic proportions of the human gestalt - changing proportions for the human form in fine art.
3. Combination types to index 12 - examination of melodic moments within each index (counterpoint).
VI. Selections.
A. Scales - a study of 23 scales from diatonic, chromatic to 19-tone scale with emphasis on comparing the well tempered system.
B. Chords -
1. Polar geometry.
2. Major and Minor.
3. Genesis of chords within the partial tone diagram - interval and chord construction.
C. Melodic and chordal examples.
D. Cadence - step-dialectic - a parallel to traditional harmony.
E. Counterpoint - reciprocals and a comparison to drama. Traditional counterpoint and the partial tone system.
F. Direction - linear, polar vectors.
1. Fourier theorem.
2. A discussion of causality.
G. Interval exponents and constants.
1. Successions of same interval or same tone.
2. Color analysis.
H. Symmetry
1. Harmonic.
2. Organic.
3. Artistic - Beethoven's 5th.; examples from other disciplines.
I. Time and Space.
1. Harmonic view - string length/frequency, perspective/equal distance, major/minor.
2. Lorenz contraction, Einstein, and philosophic viewpoints.
J. Enharmonic - study of tone proportions projected into the space of an octave.
1. Interval exponential rows.
2. Syntonic Comma and Pythagorean Apotome.
K. Temperament - concept of compromise extended to octaves with less or greater than 12 tones.
L. Number Symbology - methods of harmonic number analysis.
M. Harmonics examples.
1. Norms.
2. Hierarchy.
3. Tolerance.
4. Harmonic cosmology.
• • •
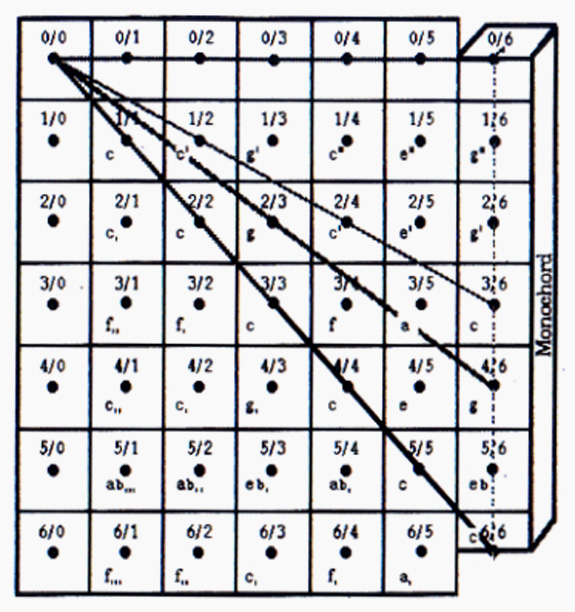
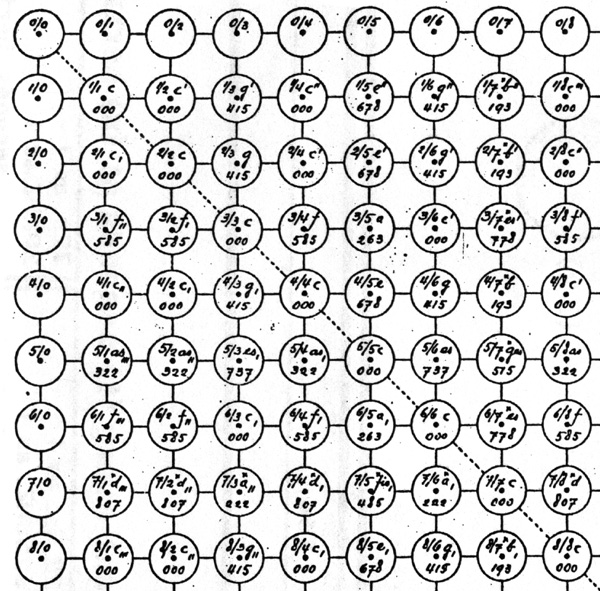
Shown above is the Lambdoma tone value and tone number diagram with an attached monochord. It is here limited to an index of 6, but the Harmonic Index is actually unlimited depending upon the application. This is quite probably what Plato was referring to in the Phaedrus when Socrates renounces the dialog of Lysias. 'Would they not treat him as a musician would treat a man who thinks he is a harmonist because he knows how to pitch the highest and lowest note, because he who would be a harmonist must certainly know this, and yet he may understand nothing of harmony [sic], music.' That is the point where tone takes on the attribute of data and communication. Note the use of irrational numbers on the top and left side of the diagram. The Greeks labeled the 0/0 as Eidos, that translates as God or Out of Nothing. This point is known as the origin and is the point from which the Harmonic Cosmology emanates in multidimensions. All values originate in this point. Without this origin the monochord on the right could not be cut perfectly by the ratios in the table, when using only those ratios to the right of the daigonal to cut the string length. From left to right the tonal progression is the overtone row and from top to bottom the undertone row. This cross-hatching of overtones and undertones forms the ratios that comprise the Lambdoma. Both major and minor (musical) universes are in evidence here. Below is an Index 8 Lambdoma table that also shows the 3 place logarithm associated with each harmonic.
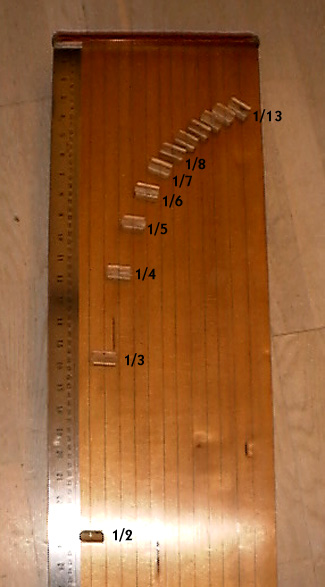
Below are two pictures of a 1200 mm. monochord. Actually this monochord has 13 strings, but they are all tuned to the same pitch. On each monochord the tiny bridges are set to the measured tones of the overtone series.

The reciprocity of string length to frequency is as follows. Cutting a string in half (1/2) doubles the frequency (2/1). Doubling a string length (2/1) divides the frequency in half (1/2). String length is the spatial element and frequency is the time element. Either can be taken as the basis for the Lambdoma. When string length is used as its basis the tonal character is a major tonality, frequency is equidistant (octaves) that expand and string length is in perspective (overtone series) that contract. When frequency is used as its basis the tonal character is minor, the string length is equidistant (octaves) that expand and the frequency is in perspective (undertone series) that contract.
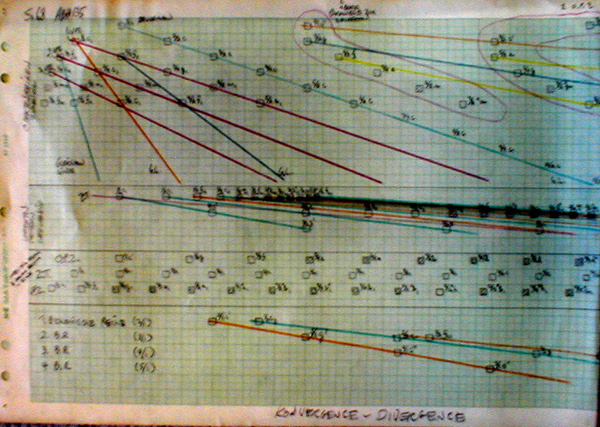
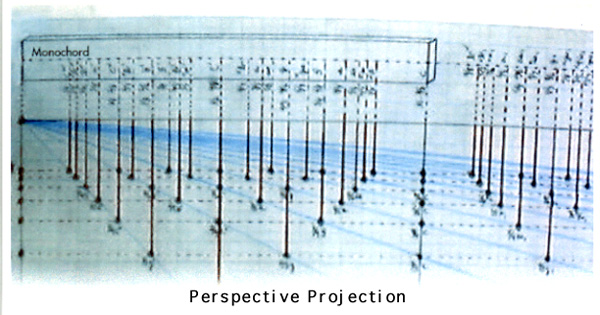

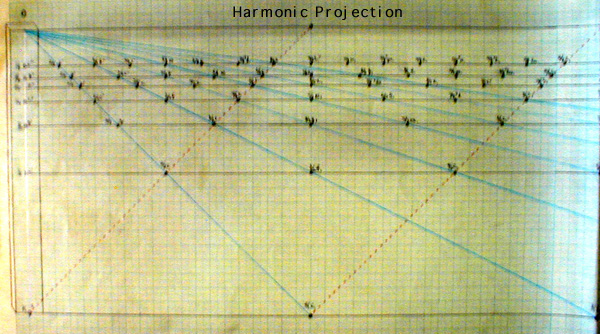
Below is a numbered diagram for the Lambdoma. Color unifies pitch-ratio that are the same. An individual color also shows the rays of same pitch that emanate from the origin.
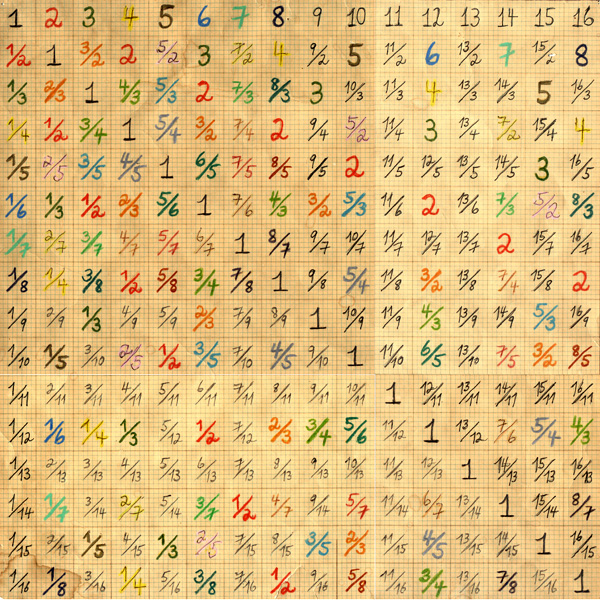
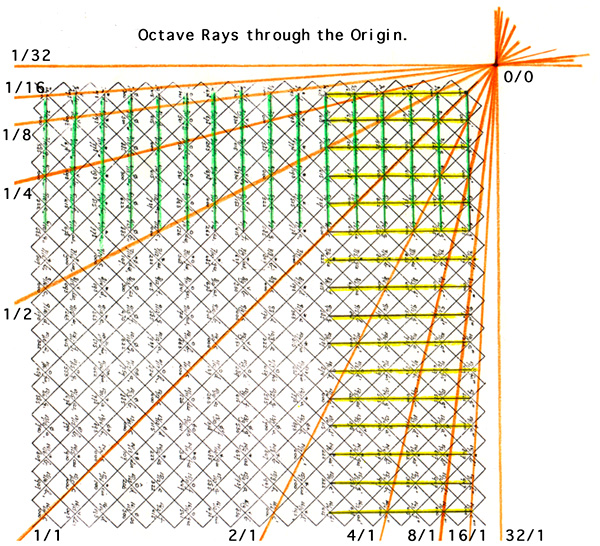

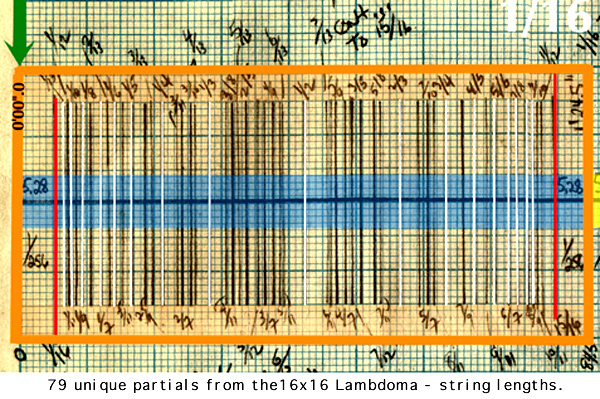
The full 16X16 Lambdoma contains 256 parts, but only 79 of them are unique. The other 177 parts are repetitions in the Harmonics. As the index increases the number of unique parts change in a very interesting manner, but that is beyond the scope of this introduction.

The following diagrams trace the primary intervals through the Lambdoma.
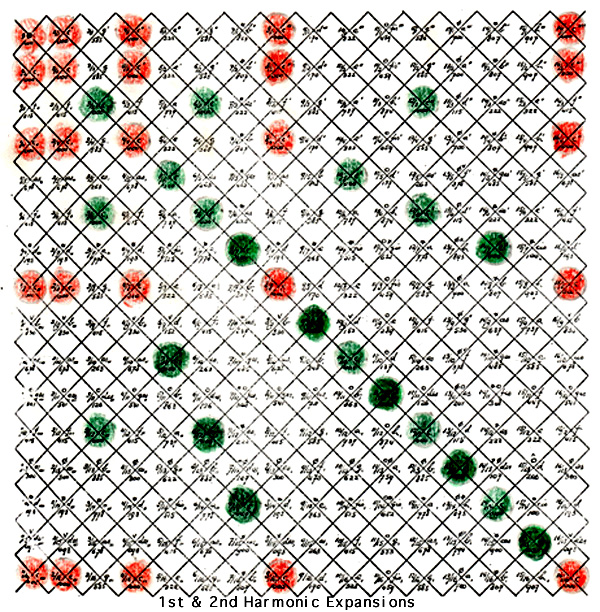
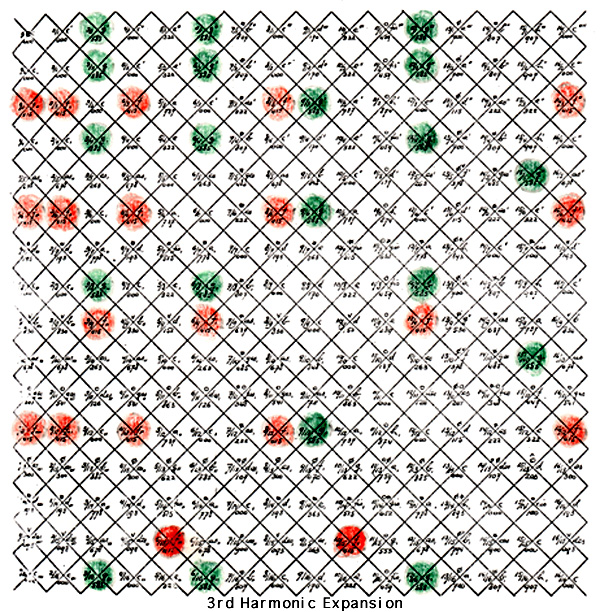
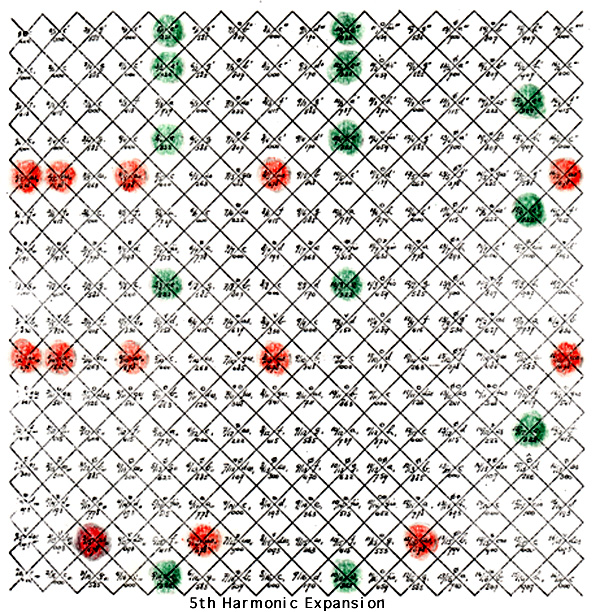



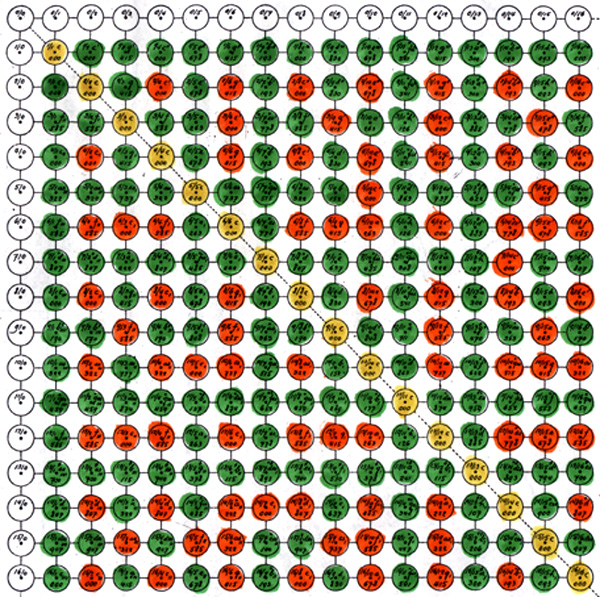
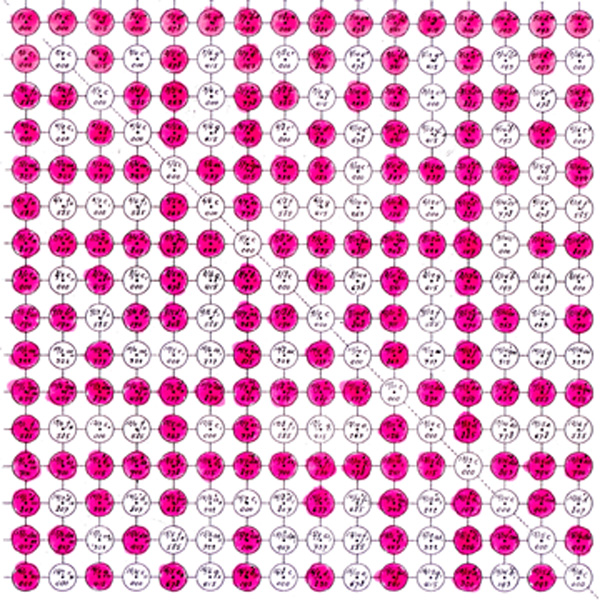
Color drop Lambdoma diagrams:

Some harmonic projections from geometry:
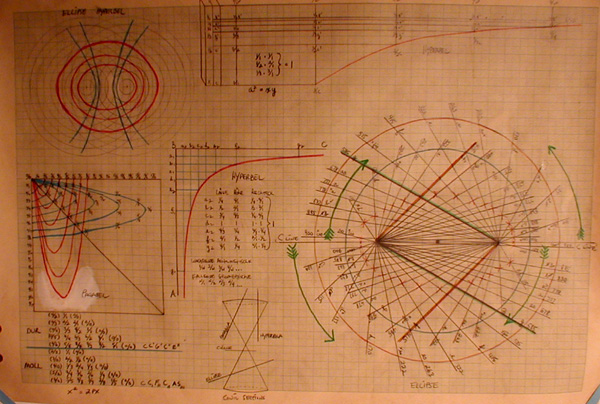
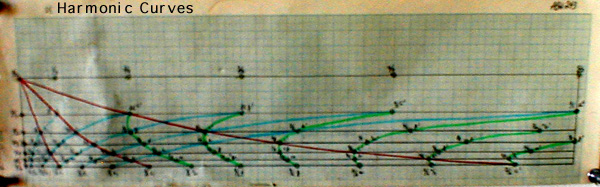
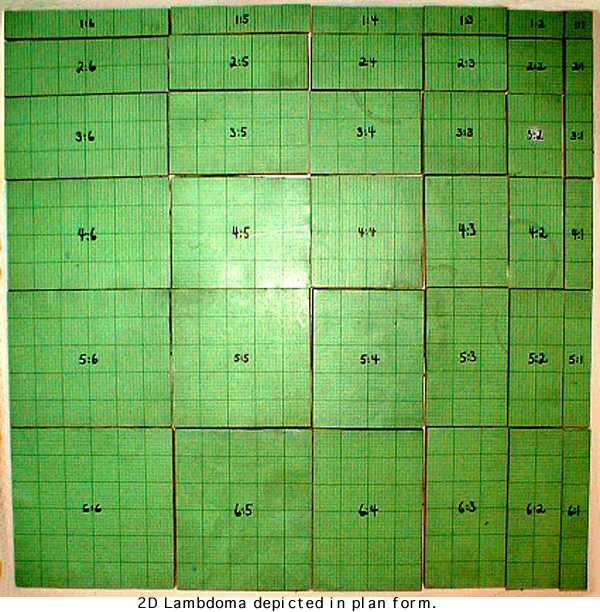
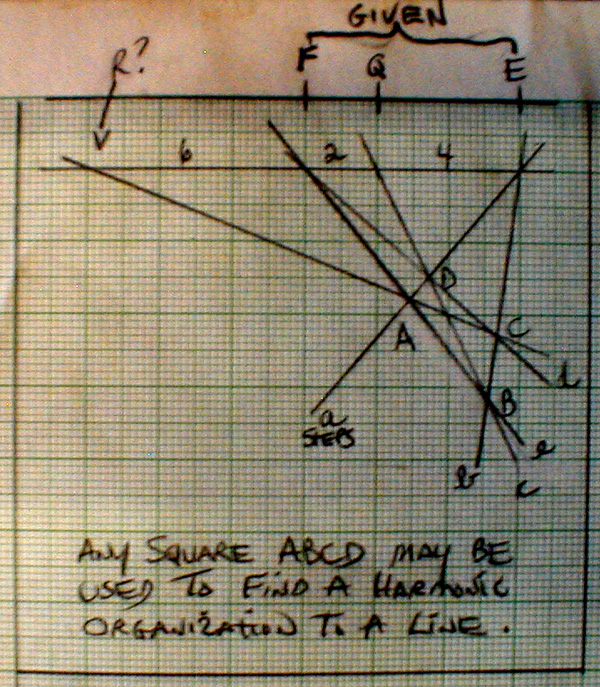
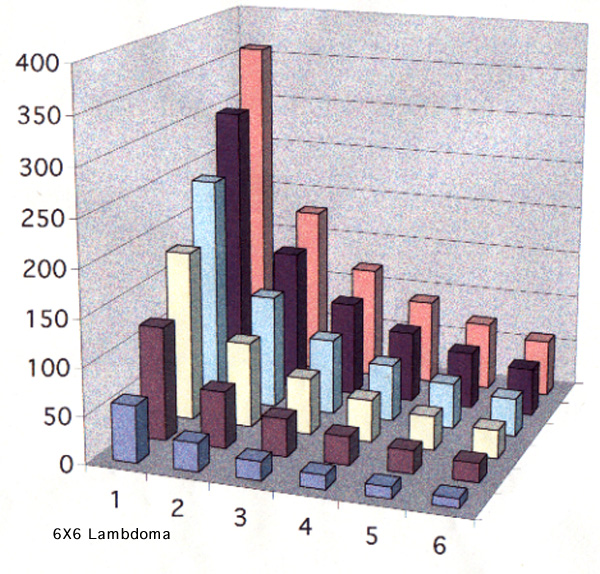
As the index of the Lambdoma expands there are ever increasing numbers of new ratios. This expansion fills a macro and micro universe. Sonically the pitches of the Lambdoma quickly reach beyond the range of hearing in both realms. These frequencies can still be transposed back into a base octave within the range of hearing and be heard. For sounding, the extent of the index is dependent upon the resolution of current frequency generators. Otherwise the index can reach into the nano-world as well as the cosmos.
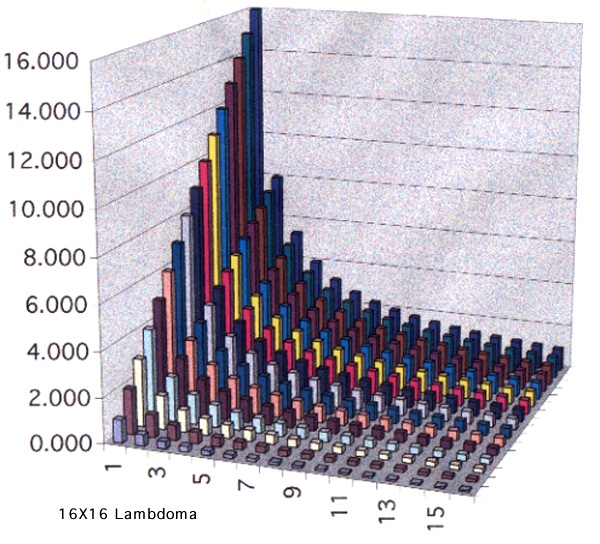
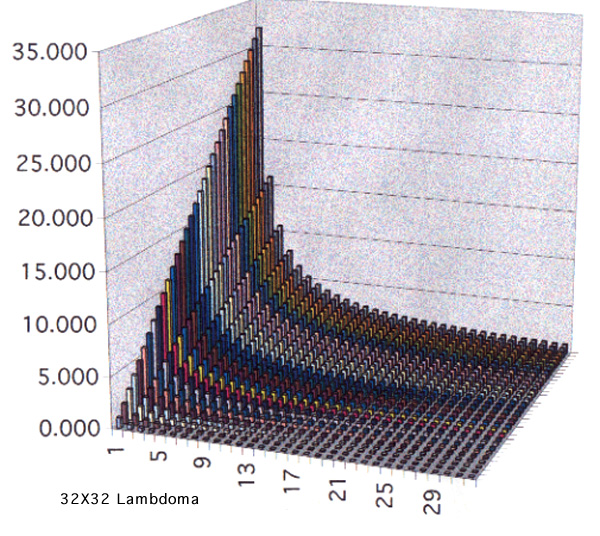
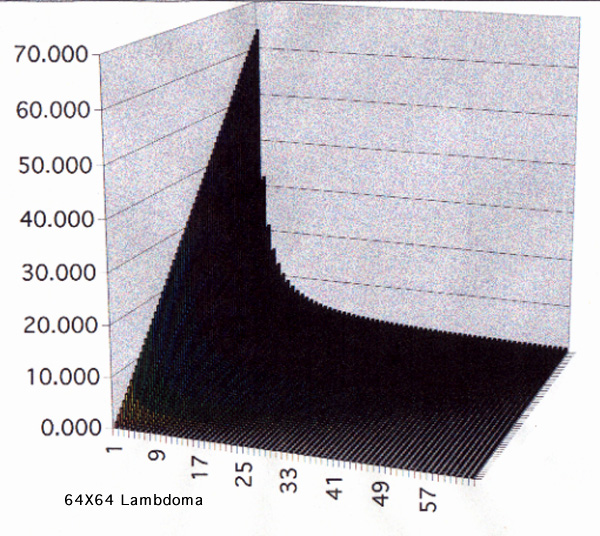
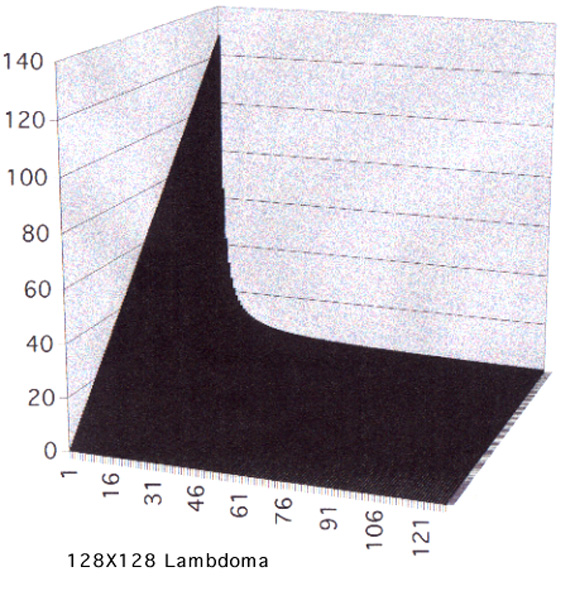
Below is a spread sheet reduction print out of Lambdoma numbers for index 128. This index has 16,384 ratios. There is a correlation here with the work of the mathematician Stephen Wolfram and his theory of cellular automata. In spite of this bad print out, if you scroll down through the index 128 you can see tonal rays emanating from the origin in the top right corner. Observe that blank spaces also move in the fashion of cellular automata. The Lambdoma is one historical reference for Stephen Wolfram's A New Kind of Science - Wolfram Media 2002.

I like to imagine the Lambdoma as a tool to examine the audio spectrum. If each box in its matrix were a light emitting diode then playing sounds into it would light the source spectrum. Harmonics is a great tool for realizing compositional ideas. Harmonics can be applied to many disciplines by those who would enjoy seeing and hearing, as a tool, for interdisciplinary communication. Harmonics can be taught in an open forum based upon interest. Initial induction to Harmonics would best be applied at a Music Department because of the sound factor, but its study should remain elective to an entire University. After initiation, the Harmonics lecture can move freely between departments utilizing whatever specific resources each department might provide. Practicing tones on the green, sight and sound movement are yet other opportunities for harmonists to gather together.




I wish you enjoy this short introduction. I have prepared lectures and demonstrations on the monochord where all of the harmonic theorms are brought to light that enable participants to become harmonists.

© Drew Lesso 2007
Hits to this Page: 4263
Hits to this Page: 4263